Do you know why a spring compresses or expands when you apply force on it? It’s the elastic property and flexibility that helps springs to behave like that. Hooke’s law is based on this theory. Let us know more about Hooke’s law in this article and learn about Hooke’s law formula, equation, and some examples. So Let’s dive in.
What Is Hooke’s Law?
Hooke’s law states that for a small amount of deformation of an object, the amount of deformation is directly proportional to the deforming force or load. The deforming load can be an extension, compression, bending, stretching, squeezing, etc. Hooke’s law works well only when the object is in the elastic zone. That means that if you withdraw the force, the object will come back to its original shape.
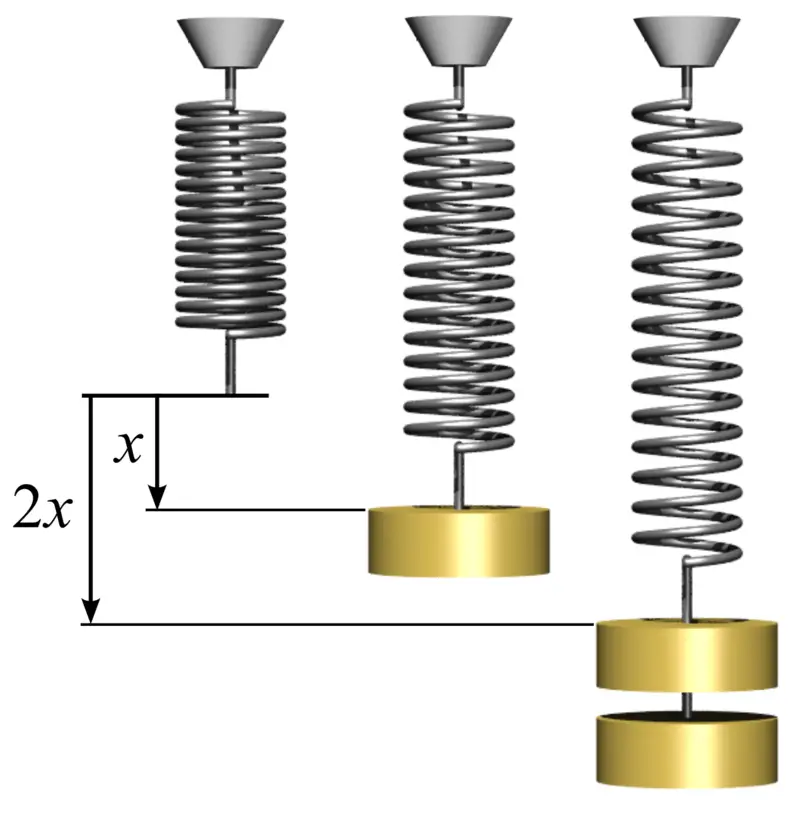
Hooke’s law can also be explained by the fact that the displacement of each molecule, atom, and ion of an object is directly proportional to the force applied. Hooke’s law is ideally suited for objects having spring-like behavior. But it can also be applied to any other solid body
The Hooke’s is discovered by the great English scientist Robert Hook in 1660
You may like to read: What is Poisson’s Ratio
Hooke’s Law formula: Hooke’s law equations
Let us consider that the force applied on an object is F and the amount of displacement is X, then the Hooke’s law can be explained as F=kX, where k is a constant whose value depends not only on the elastic properties of materials but also on the applied force and displacement.
Hooke’s Formula, F=kX or F=-kX
Hooke’s law can also be expressed as F=-kX. The reason for adding the minus symbol is that even the resultant restoring force ( Equal and opposite direction of applied force) is directly proportional to the amount of displacement.
Both the formula is applicable only when the object is capable of coming back to its original shape after removing the force. If more force is applied, the object will go into the plastic zone. In that, the force won’t be proportional to the amount of displacement.
Hooke’s law graph
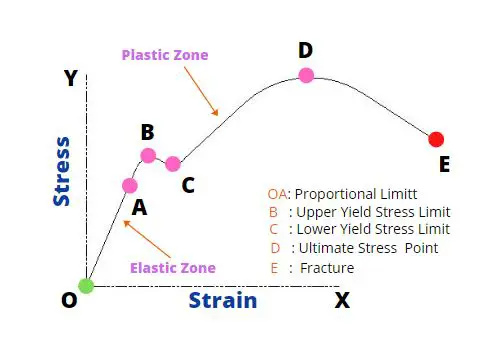
If you look into the stress-strain curve of the above object, Hooke’s law is applicable only in-between point O-A. This is the zone where strain behaves linearly with stress and this is called the elastic zone. If more load is applied above the elastic limit, the object starts to get into the plastic zone, which means that the object can not go back to its original shape if the load is removed.
You may like to Read: What is Young’s Modulus
Unit of Hooke’s Law
In the English system, the unit of measurement of Hooke’s law is Pascals and in the metric system, the unit of measurement is Newton per meter (N/m)
Usage of Hooke’s Law
Hooke’s law can be applied to all materials which come back to original shape upon withdrawal of the load. It is applicable to all materials which follow the linearity of stress and strain in the elastic limit.
Any object made of steel or aluminum or any other metals that follows the stress-strain linearity in the elastic zone uses Hooke’s law. Material such as rubber or any plastic does not hold good with Hooke’s law as the amount of displacement gets changed due to other factors like temperature and material composition.
Hooke’s Law example
Let us solve this quick task of calculating the Hooke’s Law constant
Q. An object is displaced by 100 mm with a force of 400N. What is the Hooke’s constant for the object?
In the above case, the force is in metric format. So let us first convert the displacement into the metric format
100 mm=.1 m
As we know the formula for Hooke’s is, F=kX, So in this case k would be equal to F/X
k=F/X= 400/0.1= 4000
So the Hooke’s constant in the above case is 4000 N/m
You may like to read: What is Bulk Modulus
Conclusion
Hooke’s law or the Hooke’s constant is a key value to determine the flexible properties of spring and other similar objects. Hook’s constant gives us information about how much would be displacement with a given amount of force. It also gives insights about when the part may land into the plastic zone and eventually fails.
That’s all I have in this article. I hope you could able to get some basic ideas about Hooke’s law from this article. If you have any queries or questions, please do write in the comment section and I will be happy to respond.
Frequently Asked Questions ( FAQ)
What is Hooke’s Law
Hooke’s law states that for a small deformation of an object, the amount of deformation is directly proportional to the deforming force or load
What is the Hooke’s law formula
Hooke’s law formula can be expressed as F=kX, where F is the applied force, X is the displacement and k is the Hooke’s constant.